Mathematics Questions and Answers - Form 2 Term 3 Opener Exams 2023
Get the complete Mathematics Questions and Answers - Form 2 Term 3 Opener Exams 2023 PDF on WhatsApp by tapping on the button
INSTRUCTIONS TO CANDIDATES
- This paper consists of two sections: Section I and Section II.
- Answer all questions in section I and Section II.
- Show all the steps in your calculations, giving your answers at each stage in the spaces below each question.
- Marks may be given for correct working even if the answer is wrong.
- KNEC Mathematical tables may be used.
SECTION I (50 Marks)
Answer all the questions in the spaces provided
- Find the possible values of x in the equation. (3 marks)
9x = 27(2x+12) - Express
in the form a/b where a and b are constants hence find the difference between a and b. (3 marks)
- A trader buys 200 items at a total cost of shs 6000 .She sells 150 of them at a profit of 25% and the remainder at a loss of 8% .
- Calculate the amount of net profit she made (2marks)
- Express the net profit as a percentage of the initial cost price of all items (2 marks)
- The width of a room is 8m less than length. Find the measurement of the room if its Perimeter is 48m. Hence calculate the area of the room. (3 marks)
- Use tables to evaluate √1.875+2.4232 leaving your answer correct to 2 decimal places. (2 marks)
- Given that sinθ=0.8 where is an acute angle ,find without using mathematical tables or calculator
- Cos θ (2marks)
- tan(90−θ) (1mark)
- tan θ (1mark)
- The currency exchange rates at a given bank in Kenya are as follows.
A tourist arrived in Kenya with 5000 US dollars which he converted to Kenya shillings upon arrival. He spent Ksh.214, 500 and converted the remaining to Sterling pounds. How many pounds did he receive? (3marks)Currency Buying Selling 1 Sterling Pound 135.50 135.97 1 US dollar 72.23 72.65 - Use reciprocal tables and cube tables to evaluate (3 marks)
5.23 + 4
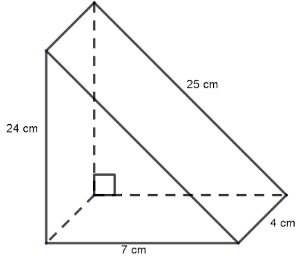
0.7052 - Find the surface area of the solid below (3 marks)
- Without using a calculator or mathematical tables simplify. (3 marks)
36 − 8 × − 4 −15 ÷ − 3
3× − 3 + − 8(6 −( −2) - An isosceles triangle has congruent sides of 20 cm. the base is 10 cm. determine the area of the triangle. (3 marks)
- Two similar containers have capacities of 1000 litres and 1728 litres respectively .Given that the smaller container has an area of 750 cm3.Calculate the surface area of the larger container. ( 3marks)
- The circle whose length is 2.2m subtends an angle of 60° at the centre. Calculate the area of the minor segment of the circle.
(Take = 22/7) (3 marks) - Find the coordinates of the point of intersection of the lines 2x − 3y + 5 = 0 and 2y − x = −3. (3 marks)
- Given that (x+60)° = cos(2x)°find tan (x+60)° (3marks)
- A piece of land is to be divided into 20 acres or 24 acres or 28 acres for farming and Leave 7acres for grazing. Determine the smallest size of such land. (3 marks)
SECTION II
- A triangle A(1,1), B(2, 4) and C(4, 2) undergoes:
- Reflection in the line y =° x. Obtain the coordinates of its image A’B’C’ after the above transformation and show both the object and the image on a Cartesian plane. (3 marks)
- On the same axis, draw A’’B’’C’’, the image of A’B’C’ after a negative quarter turn about the origin and state the coordinates of A’’, B’’ and C’’. (2 marks)
- Describe the transformation that maps triangle A’’B’’C’’ onto triangle ABC. (2 marks)
- Draw the line x = −3 and draw triangleA’’’B’’’C’’’, the image of triangle A’’B’’C’’ under a reflection in the linex = − 3. State the coordinates of triangleA’’’B’’’C’’’. (3 marks)
- A line l1 passes through the point −2,3 and −1, 6
- Find the equation of l1 in the form y = mx+c (3marks)
- Find the equation of l2 which is perpendicular to line l1 at −1, 6 in the form ax + by − c = 0 Where a,b and c are constants (3marks)
- Given that another line l3 is parallel to l1 and passes through point (1 ,2)
- Find the equation of l3 in the form y = mx + c (2marks)
- Find the x-intercept of line l3 (2marks)
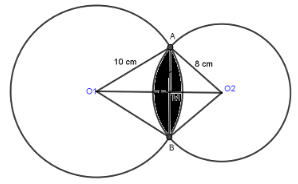
- The figure below shows two circles of radii 10cm and 8cm and centres O1 and O2 respectively. The common chord AB=12cm If O1O2 is a perpendicular bisector of AB use (π=3.142)
- Calculate correct to 2 decimal places
- angle AO1B (1mark)
- Angle AO2B (1 mark)
- Area of triangle AO1B (2marks)
- Area of triangle AO2B (2marks)
- The area of the shaded part to 4 significant figures (4 marks)
- Calculate correct to 2 decimal places
-
- The price of an article was raised by 25% and a month later the new price was lowered by 20%. What was the new price if the original price was Ksh. 750? (3 marks)
- Water flows from a tap at the rate of 27cm3/second into a rectangular container of length 60 cm, breadth 30 cm and height 40 cm. If at 5.00 p.m. the container was half full, what will be the height of water at 5.08 p.m.? (4 marks)
- Akinyi sold a mobile phone costing Ksh. 4000 at a profit. She earned a commission of 10% on the profit. Find the amount earned if she made a profit of 40%. (3 marks)
- A salesman is paid a salary of Sh. 10,000 per month .He is also paid a commission of sales above Sh 100000 .In one month he sold goods worth Sh. 500,000 .If his total earnings that month was shs 46,000
- Calculate the rate of commission (4marks)
- If the rate of commission in (a) above was reduced to 7.65 % and his monthly salary increased by sh. 1000 , find his total new earning that month for selling the same amount of goods . (4marks)
- The marked price of TV set in shop is sh.40000 Mueni bought the TV at 10% discount calculate the amount of money she paid for the TV. (2marks)
MARKING SCHEME
| NO | WORKING | MARKS | ||||||||||||||||||||||||||||
| 1. | 32x = 36x+36
2x = 6x + 36
2x − 6x = 36
−4x = 36
x = −9
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 2. | r = 12.42727 ........ (i)
10r = 124.27272 ....(ii)
100r = 1242.72727 ..... (iii)
1000r = 12427.27272 ...... (iv)
Subtract (ii) from (iv)
1000r = 12427.27272
10r = 124.27272 −
990r = 12 303
r = 12 303 990 a = 12 303, b = 990
a − b = 12 303 − 990 = 11 313
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 3. |
|
M1 A1 M1 A1 |
||||||||||||||||||||||||||||
| 4. | let width = x
length =x +8
p = 2(x+8+x) = 48
4x = 48 − 16
x = 8cm
length =16cm width = 8cm
Area = 16×8 = 128cm2
|
M1 B1 A1 |
||||||||||||||||||||||||||||
| 5. | √1.875 = 1.3693
2.4232 = 5.871
⇒ 1.3693 + 5.871 = 7.2403
≅ 7.24 (2 d.p)
|
M1 A1 |
||||||||||||||||||||||||||||
| 6. |
|
M1 A1 A1 B1 |
||||||||||||||||||||||||||||
| 7. | Ksh.5000 x 72.23 = Ksh.361,150
Remainder after expenditure
361,150 = 214,500 = Ksh.146,650
No. of £ = 146650 × 1
135.97 = £1078.55
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 8. |
5.23 = 140.61
4 = 4 × 10 × 0.1418 = 5.672
0.7052 ⇒ 140.61 + 5.672 = 146.282
|
M1A1 |
||||||||||||||||||||||||||||
| 9. |
1/2 × 7 × 24 × 2 = 128cm2
25 × 4 = 100cm2
7 × 4 = 28cm2
24 × 4 = 96cm2
total surface area = 168 + 100 + 28 + 96
= 392cm2
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 10. |
N
36 − 8× − 4 −15 ÷ −3
36 − 8 × − 4 + 5
36 + 32 + 5 = 73
D
3 × −3 + −8(6−(−2)
−9 − 64 = −73
N/D = 73 = −1
−73 |
M1
|
||||||||||||||||||||||||||||
| 11. | s = 0.5(10 + 20 + 20) = 25
A = √(25(25−10)(25−20)(25−20))
= √(25×15×5×5)
= 96.82 cm2 (4 s.f)
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 12. |
V.S.F = 1728 = 216
1000 125 L.S.F= ³√(216/125)
= 6/5
A.S.F= (6/5)2 = 36/25
Area of larger container = 36/25 × 750 = 1080
|
M1 A1 |
||||||||||||||||||||||||||||
| 13 | L = ∅/360 × 2r
2.2 = 60 x 2 x 22 x r
360 7
r = 2.2 x 360 x 7
60 x 44
r = 2.1 60 × 22 × 2.12 − 1/2 × (2.1×2.1× sin 60)
360 7 2.23 − 1.9095 = 0.4004
|
M1 M1 A1 |
||||||||||||||||||||||||||||
| 14. |
2x − 3y = −5 .... (i)
2y − x = −3 ........(ii)
From (ii), x = 2y + 3
2(2y+3) − 3y = −5
4y + 6 − 3y = −5
y = −11
x = 2 (−11) + 3 = −19
Coordinates are (−19, −11)
|
M1
B1 |
||||||||||||||||||||||||||||
| 15. | x + 60 + 2x = 90
3x = 30
x = 10°
Tan 70 = 2.747
|
M1 B1 A1 |
||||||||||||||||||||||||||||
| 16. |
840 + 7 = 847
|
B1 |
||||||||||||||||||||||||||||
| 17. |
|
B1
B1 reflection on y=-x
B1 ∆A'B'C'
B1 rotation
B1 ∆A''B''C''
B1 B1
B1
B1 reflection on x=-3
B1 ∆A'''B'''C'''
|
||||||||||||||||||||||||||||
| 18. |
|
M1 M1 A1 B1 M1 A1 M1 A1 M1 A1 |
||||||||||||||||||||||||||||
| 19. |
|
A1 |
||||||||||||||||||||||||||||
| 20. |
|
M1 M1A1 M1 M1 M1 A1 M1 M1 A1 |
||||||||||||||||||||||||||||
| 21 |
|
B1 M1 B1 A1 M1 M1 B1 A1 M1 A1 |